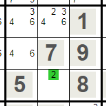
 Naked Single: According to Sudoku rules, the number in a cell can appear only once in each block, each row, and each column. For each cell we can create a list of possible candidates that can appear in a cell. Read More
|
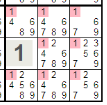 Conflicting Candidates: A list of hints in any cell represents the variety of possible candidates for this cell. If one of the possible candidates in the cell (a hint) has the same value as any cell in the same box, row, or column, Read More
|
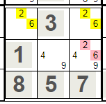 Naked Pairs: Naked Pairs are the two cells in the same block, row, or column which have exactly the same pair of two candidates and there are no other candidates in these two cells. Read More
|
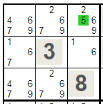 Hidden Singles: According to Sudoku rules, each number should appear at list once in each box, row, and column. Hidden Single is a number that appears as a possible candidate Read More
|
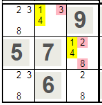 Hidden Pairs: Hidden Pairs are similar to the Naked Pairs. They are two cells in the same block, row, or column which have exactly the same pair of two candidates. Read More
|
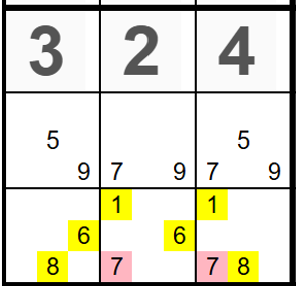 Hidden Triples: Hidden Triples are very similar to Hidden Pairs. Hidden Triples involve three candidates located in only three cells within the same 3x3 square, row, or column. Read More
|
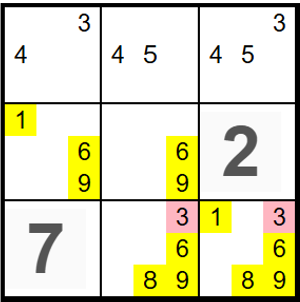 Hidden Quads: The Hidden Quads Sudoku patterns are similar to the Hidden Triples. As the name suggests, Hidden Quads have four candidates located in four cells. Read More
|
 Box/Line Reduction: The Box/Line Reduction technique is similar to the Hidden Single. In the Hidden Single technique, we are looking for a number that appears as a possible Read More
|
 Naked Triples and Quads: Naked Triples are very similar to Naked Pairs. As the name indicates, there is a list of three candidates, and are three cells in the same block, row, or column, which have no other candidates in these three cells except those from the list. Unlike Naked Pairs, it is not required for Naked Triples that all three sells have all tree candidates, but each of selected candidates should be presented in at least one of these cells. Read More
|
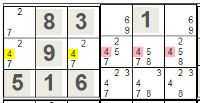 Pointing Pairs: The Pointing Pairs technique helps to remove conflicting candidates in the same row or column. If a number appears as a possible candidate only in one row or column in a box, this number should not appear in the specified row or column in any other box. Read More
|
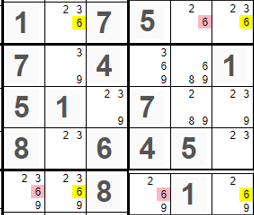 X Wing: The X-wing is an advanced Sudoku technique that uses single hints in two parallel rows or two parallel columns, forming an X pattern. Read More
|
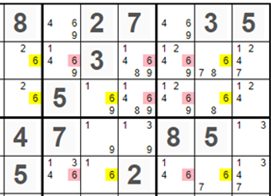 Swordfish: The Swordfish sudoku pattern is an extension of the X-Wing pattern. The Swordfish sudoku pattern is formed by the same hint appearing in nine cells at the crossing of three rows and three columns. Read More
|
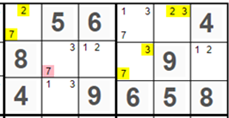 Y Wing: The Y-Wing Sudoku pattern is reminiscent of the X-Wing pattern, as it also consists of four interrelated cells. Unlike the X-Wing pattern, the four cells in a Y-Wing pattern do not necessarily form a rectangle. Read More
|
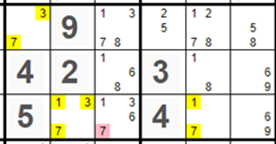 XYZ Wing: The XYZ-Wing is an advanced Sudoku-solving technique, extending the Y-Wing technique. It involves three cells that contain only three possible values. Read More
|
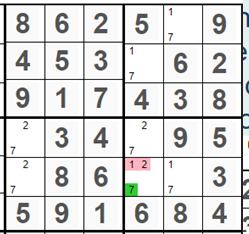 Bivalue Universal Grave (BUG): The Bivalue Universal Grave is a Sudoku-solving technique based on the assumption that a Sudoku puzzle, where all remaining cells contain just two candidates, does not have a unique solution. Read More
|
 Unique Rectangles: The Unique Rectangles technique assumes that a Sudoku puzzle has only one solution. Any configuration that could lead to multiple solutions is considered invalid. Read More
|
 Singles Chains: The Singles Chains sudoku-solving strategy is an advanced and powerful technique. Sometimes this technique is also called Single Coloring. It goes through the chain of single interrelated candidates in different cells and color mutually exclusive candidates in two different colors. Read More
|
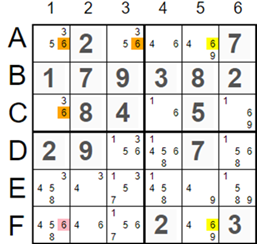 Rectangle Elimination: The Rectangle Elimination Sudoku solving pattern allows to eliminate a single candidate and involves cells in two rows and two columns. Read More
|
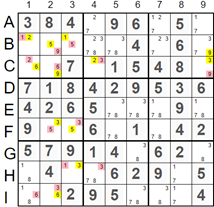 3D Medusa: The 3D Medusa Sudoku solving strategy is an extension of the Singles Chains strategy. Singles Chains link candidates that appear twice in the same column, row, or square. 3D Medusa extends this strategy by linking two candidates that appear in the same cell. Read More
|
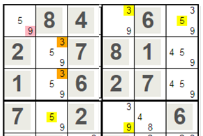 Chains: Chains are the most complicated Sudoku solving techniques. This strategy is used when no other technique allows solving the Sudoku. A Chain is a sequence of related candidates. Making an assumption on one candidate might affect other candidates in a Chain. Read More
|
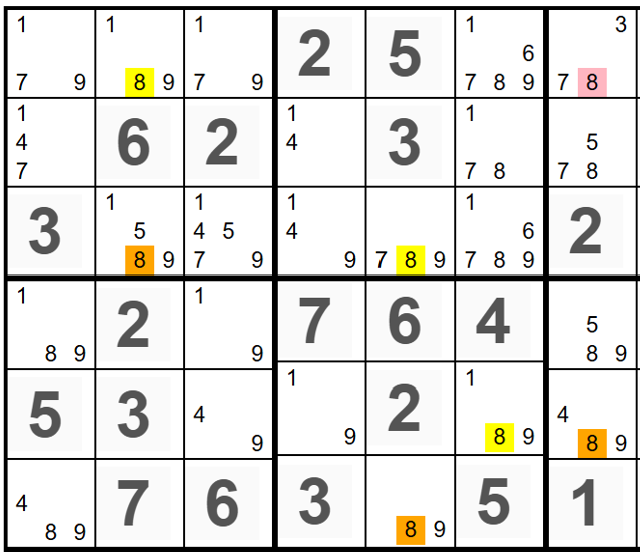 X-Chains: X-Chain is a powerful Sudoku-solving technique. It is an extension of the Simple Coloring technique that uses alternating strong and weak links. Read More
|
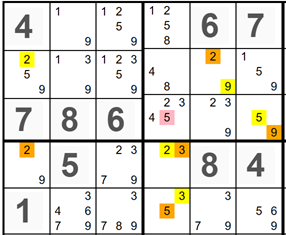 XY-Chains: The XY-Chains technique is an extension of X-Chains. In addition to the X-Chains linking technique, XY-Chains allows us to link different candidates within the same cell.. Read More
|
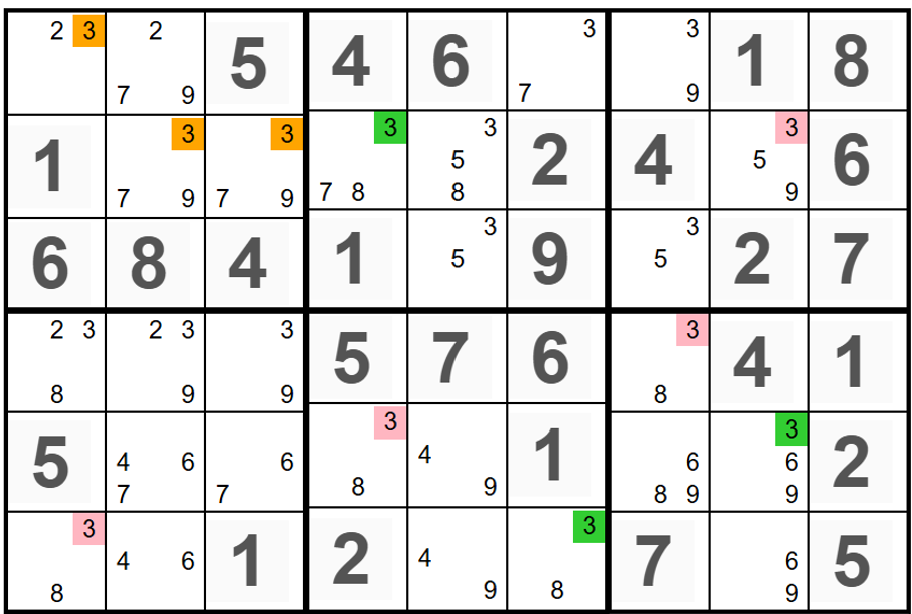 Singles Chains Elimination: The Singles Chains Elimination sudoku solving technique extends the Rectangle Elimination technique by combining it with the Singles Chains technique. Read More
|
 Medusa 3D Elimination: The Medusa 3D Elimination technique enhances the Rectangle Elimination technique approach by utilizing Medusa 3D chains. Read More
|
 X-Chains Elimination: The X-Chains Elimination sudoku solving technique extends the Rectangle Elimination technique by combining it with the X-Chains technique. Read More
|
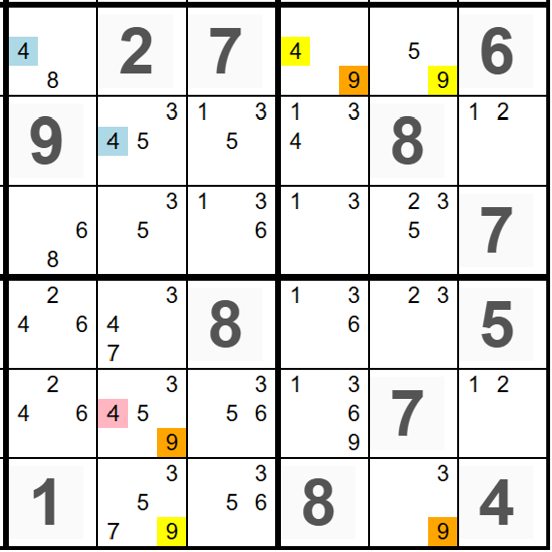 XY-Chains Elimination: The XY-Chains Elimination sudoku solving technique extends the Rectangle Elimination technique by combining it with the XY-Chains technique. Read More
|
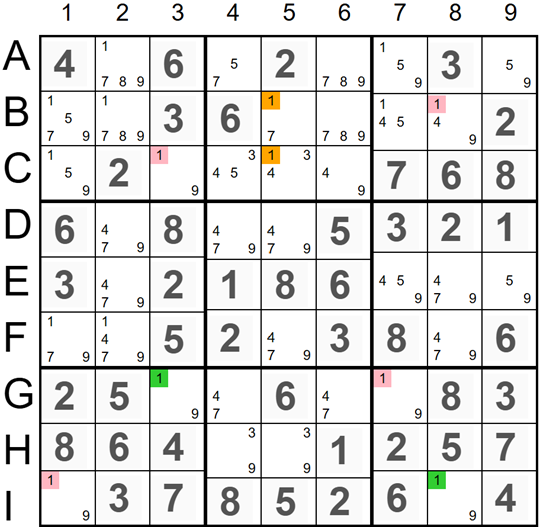 Singles Chains Parallel Elimination: The Singles Chains Parallel Elimination sudoku solving technique is similar to the Singles Chains technique. It requires finding a 3x3 square where all cells with a certain candidate are located in two rows or two columns within the 3x3 square. Read More
|
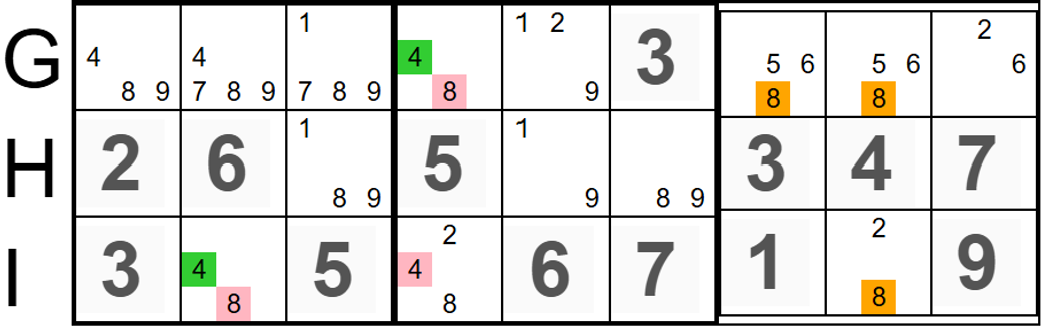 Medusa 3D Parallel Elimination: The Medusa 3D Parallel Elimination Sudoku solving technique is similar to the Medusa 3D Elimination technique. The difference between these two techniques lies in the first step. Read More
|
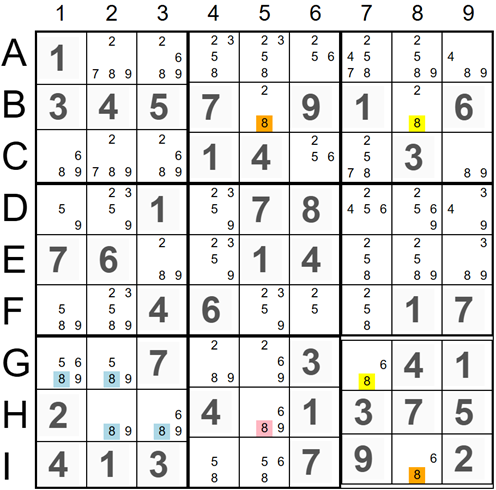 X-Chains Parallel Elimination: The X-Chains Parallel Elimination Sudoku solving technique is similar to the X-Chains Elimination technique. It works when there is a 3x3 square where all cells with a certain candidate are located in two rows or two columns. Read More
|
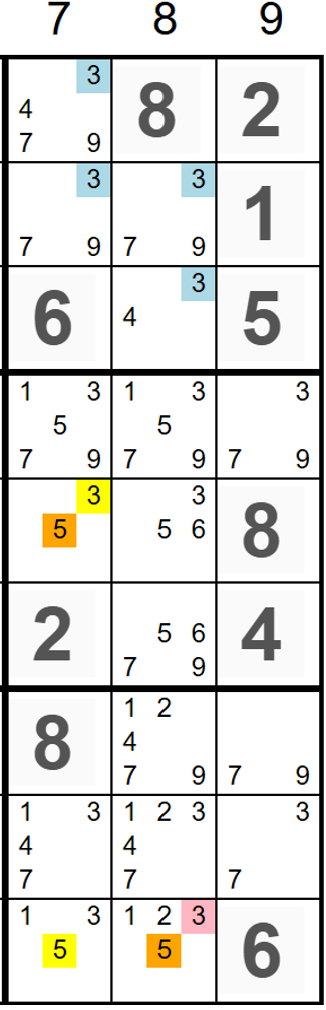 XY-Chains Parallel Elimination: The XY-Chains Parallel Elimination technique is similar to the XY-Chains Elimination sudoku solving technique. Read More
|